Leiam em: http://www.dedalus-atlas.blogspot.com/
O que faz uma pessoa tomar uma atitude dessas ? Posso citar vários motivos, mas não aceito nenhum. Desistir nunca pode ser uma opção. Ontem estava muito triste e sentido, hoje estou tremendamente chateado com este meu amigo. Estas fases as quais estou passando são esperadas quando sofremos tamanha perda.
Valeu Sandro. Valeu nossa discussões que não levavam a nada a não ser satisfazer nossos próprios egos. Valeu pelos papos de cultura inútil, mas que fazíamos rir a beça. Tá bom ... Agora só depois !!!
Abraços !!!
HULK
sexta-feira, 22 de outubro de 2010
quinta-feira, 21 de outubro de 2010
Remote desktop only on local network
Remote desktop only on local network: "From: http://ubuntuforums.org/showthread.php?t=1143079&page=2
had the same problem with 9.04, no advanced tab, server was not listening to the port (even default one), but I just found out how to set all this.
To use an alternative port:
run gconf-editor from terminal
in the open window go to:
/desktop/gnome/remote_access
Change the alternative_port value (mine was 5900) to the one you want
Select (check) use_alternative_port
And now, run:
/usr/lib/vino/vino-server
(you can make it run at login by addin the previous line in the: System->Preferences->Startup Applications)
Good Luck!
zensys
June 17th, 2009, 03:01 PM
I had the same problem but was able to solve it by unchecking the option 'configure network automatically to accept connections' This option requires the UPnP feature in your router to be enabled.
Surprisingly (I find computers have a strong resemblance to women) now I also have outside connectivity with the automatically accept connections option checked and UPnP disabled.
Hope this helps more than it confuses!"
had the same problem with 9.04, no advanced tab, server was not listening to the port (even default one), but I just found out how to set all this.
To use an alternative port:
run gconf-editor from terminal
in the open window go to:
/desktop/gnome/remote_access
Change the alternative_port value (mine was 5900) to the one you want
Select (check) use_alternative_port
And now, run:
/usr/lib/vino/vino-server
(you can make it run at login by addin the previous line in the: System->Preferences->Startup Applications)
Good Luck!
zensys
June 17th, 2009, 03:01 PM
I had the same problem but was able to solve it by unchecking the option 'configure network automatically to accept connections' This option requires the UPnP feature in your router to be enabled.
Surprisingly (I find computers have a strong resemblance to women) now I also have outside connectivity with the automatically accept connections option checked and UPnP disabled.
Hope this helps more than it confuses!"
segunda-feira, 4 de outubro de 2010
Método de integração
Método de integração: "
If the integrand contains a single factor of one of the forms we can try a trigonometric substitution.
we can try a trigonometric substitution.
If the integrand contains a piece of the form we use the substitution
we use the substitution
This will transform the integrand to a trigonometic function. If the new integrand can't be integrated on sight then the tan-half-angle substitution described below will generally transform it into a more tractable algebraic integrand.
Eg, if the integrand is √(1-x2),
If the integrand is √(1+x)/√(1-x), we can rewrite it as
Then we can make the substitution
When the integrand contains a piece of the form we use the substitution
we use the substitution
E.g, if the integrand is (x2+a2)-3/2 then on making this substitution we find
If the integral is
then on making this substitution we find
After integrating by parts, and using trigonometric identities, we've ended up with an expression involving the original integral. In cases like this we must now rearrange the equation so that the original integral is on one side only
As we would expect from the integrand, this is approximately z2/2 for large z.
If the integrand contains a factor of the form we use the substitution
we use the substitution
Find
![\begin{matrix} \int_1^z \frac{\sqrt{x^2-1}}{x}dx & = & \int_1^\alpha \frac{\tan \theta }{\sec \theta }\sec \theta \tan \theta \,d\theta & z>1 \\ & = & \int_0^\alpha \tan^2 \theta \, d\theta & \alpha = \sec^{-1} z \\ & = & \left[ \tan \theta -\theta \right]_0^\alpha & \tan \alpha = \sqrt{\sec^2 \alpha -1} \\ & =& \tan \alpha -\alpha & \tan \alpha = \sqrt{z^2-1} \\ & =& \sqrt{z^2-1} - \sec^{-1} z & \\ \end{matrix}](http://upload.wikimedia.org/math/1/a/d/1ad57fbdf52745e23a90f03a3b87c50c.png)
Find

We can now integrate by parts
![\begin{matrix} \int_1^z \frac{\sqrt{x^2-1}}{x^2}dx & = & -\left[ \tan \theta \cos \theta \right]_0^\alpha + \int_0^\alpha \sec \theta \, d\theta \\ & = & -\sin \alpha +\left[ \ln (\sec \theta + \tan \theta ) \right]_0^\alpha \\ & = & \ln (\sec \alpha + \tan \alpha ) - \sin \alpha \\ & = & \ln (z+ \sqrt{z^2-1} ) - \frac{\sqrt{z^2-1}}{z}\\ \end{matrix}](http://upload.wikimedia.org/math/2/e/7/2e71e717c7736900735ff7672643e7ef.png)
Navigation: Main Page · Precalculus · Limits · Differentiation · Integration · Parametric and Polar Equations · Sequences and Series · Multivariable Calculus & Differential Equations · Extensions · References
Retrieved from 'http://en.wikibooks.org/wiki/Calculus/Integration_techniques/Trigonometric_Substitution'
Categories: Books to be merged | Calculus (book)
From Wikibooks, the open-content textbooks collection Calculus | Integration techniques
Jump to: navigation,| A Wikibookian suggests that Solving Integrals by Trigonometric substitution be merged into this book or chapter. Discuss whether or not this merger should happen on the discussion page. |
| ← Integration techniques/Partial Fraction Decomposition | Calculus | Integration techniques/Tangent Half Angle → |
| Integration techniques/Trigonometric Substitution |
If the integrand contains a single factor of one of the forms
 we can try a trigonometric substitution.
we can try a trigonometric substitution.
- If the integrand contains
let x = asinθ and use the identity 1 − sin2θ = cos2θ.
- If the integrand contains
let x = atanθ and use the identity 1 + tan2θ = sec2θ.
- If the integrand contains
let x = asecθ and use the identity sec2θ − 1 = tan2θ.
[edit] Sine substitution
If the integrand contains a piece of the form
 we use the substitution
we use the substitutionThis will transform the integrand to a trigonometic function. If the new integrand can't be integrated on sight then the tan-half-angle substitution described below will generally transform it into a more tractable algebraic integrand.
Eg, if the integrand is √(1-x2),
If the integrand is √(1+x)/√(1-x), we can rewrite it as
Then we can make the substitution
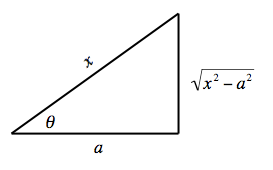
[edit] Tangent substitution
When the integrand contains a piece of the form
 we use the substitution
we use the substitutionE.g, if the integrand is (x2+a2)-3/2 then on making this substitution we find
If the integral is
then on making this substitution we find
After integrating by parts, and using trigonometric identities, we've ended up with an expression involving the original integral. In cases like this we must now rearrange the equation so that the original integral is on one side only
As we would expect from the integrand, this is approximately z2/2 for large z.
[edit] Secant substitution
If the integrand contains a factor of the form
 we use the substitution
we use the substitution[edit] Example 1
Find

![\begin{matrix} \int_1^z \frac{\sqrt{x^2-1}}{x}dx & = & \int_1^\alpha \frac{\tan \theta }{\sec \theta }\sec \theta \tan \theta \,d\theta & z>1 \\ & = & \int_0^\alpha \tan^2 \theta \, d\theta & \alpha = \sec^{-1} z \\ & = & \left[ \tan \theta -\theta \right]_0^\alpha & \tan \alpha = \sqrt{\sec^2 \alpha -1} \\ & =& \tan \alpha -\alpha & \tan \alpha = \sqrt{z^2-1} \\ & =& \sqrt{z^2-1} - \sec^{-1} z & \\ \end{matrix}](http://upload.wikimedia.org/math/1/a/d/1ad57fbdf52745e23a90f03a3b87c50c.png)
[edit] Example 2
Find


We can now integrate by parts
![\begin{matrix} \int_1^z \frac{\sqrt{x^2-1}}{x^2}dx & = & -\left[ \tan \theta \cos \theta \right]_0^\alpha + \int_0^\alpha \sec \theta \, d\theta \\ & = & -\sin \alpha +\left[ \ln (\sec \theta + \tan \theta ) \right]_0^\alpha \\ & = & \ln (\sec \alpha + \tan \alpha ) - \sin \alpha \\ & = & \ln (z+ \sqrt{z^2-1} ) - \frac{\sqrt{z^2-1}}{z}\\ \end{matrix}](http://upload.wikimedia.org/math/2/e/7/2e71e717c7736900735ff7672643e7ef.png)
Navigation: Main Page · Precalculus · Limits · Differentiation · Integration · Parametric and Polar Equations · Sequences and Series · Multivariable Calculus & Differential Equations · Extensions · References
Categories: Books to be merged | Calculus (book)
Assinar:
Postagens (Atom)




![\begin{matrix} \int_0^a \frac{1+x}{\sqrt{1-x^2}} dx & = & \int_0^\alpha \frac{1+\sin \theta}{\cos \theta} \cos \theta \, d\theta & 0 <a < 1 \\ & = & \int_0^\alpha 1+ \sin \theta \, d\theta & \alpha = \sin^{-1} a \\ & = & \alpha + \left[ - \cos \theta \right]_0^\alpha & \\ & = & \alpha + 1 - \cos \alpha & \\ & = & 1+ \sin^{-1} a - \sqrt{1-a^2} & \\ \end{matrix}](http://upload.wikimedia.org/math/2/9/9/29982769946b62e7bcf2ad9f4af5cd48.png)


![\begin{matrix} \int_0^z \left( x^2+a^2 \right)^{-\frac{3}{2}}dx & = & a^{-2} \int_0^\alpha \cos \theta \, d\theta & z>0 \\ & = & a^{-2} \left[ \sin \theta \right]_0^\alpha & \alpha = \tan^{-1} (z/a) \\ & = & a^{-2} \sin \alpha & \\ & = & a^{-2} \frac{z/a}{\sqrt{1+z^2/a^2}} & = \frac{1}{a^2} \frac{z}{\sqrt{a^2+z^2}} \\ \end{matrix}](http://upload.wikimedia.org/math/f/4/d/f4d92919d07a5d70b723f3835287c696.png)

![\begin{matrix} I & = & a^2 \int_0^\alpha \sec^3 \theta \, d\theta & & & \alpha = \tan^{-1} (z/a) \\ & = & a^2 \int_0^\alpha \sec \theta \, d\tan \theta & & & \\ & = & a^2 [ \sec \theta \tan \theta ]_0^\alpha & - & a^2 \int_0^\alpha \sec \theta \tan^2 \theta \, d\theta & \\ & = & a^2 \sec \alpha \tan \alpha & - & a^2 \int_0^\alpha \sec^3 \theta \, d\theta & + a^2 \int_0^\alpha \sec \theta \, d\theta \\ & = & a^2 \sec \alpha \tan \alpha & - & I & + a^2 \int_0^\alpha \sec \theta \, d\theta \\ \end{matrix}](http://upload.wikimedia.org/math/5/5/5/555f685884835c06e12375684d1d0f41.png)
![\begin{matrix} I & = & \frac{1}{2}a^2 \sec \alpha \tan \alpha & + & \frac{1}{2}a^2 \int_0^\alpha \sec \theta \, d\theta \\ & = & \frac{1}{2}a^2 \sec \alpha \tan \alpha & + & \frac{1}{2}a^2 \left[ \ln \left( \sec \theta + \tan \theta \right) \right]_0^\alpha \\ & = & \frac{1}{2}a^2 \sec \alpha \tan \alpha & + & \frac{1}{2}a^2 \ln \left( \sec \alpha + \tan \alpha \right) \\ & = & \frac{1}{2}a^2 \left( \sqrt{1+\frac{z^2}{a^2}} \right) \frac{z}{a} & + & \frac{1}{2}a^2 \ln \left( \sqrt{1+\frac{z^2}{a^2}}+\frac{z}{a} \right) \\ & = & \frac{1}{2}z\sqrt{z^2+a^2} & + & \frac{1}{2}a^2 \ln \left(\frac{z}{a} + \sqrt{1+\frac{z^2}{a^2}} \right) \\ \end{matrix}](http://upload.wikimedia.org/math/7/8/e/78e1654a81ca86eb28500d11389255bb.png)